Amplitude: Difference between revisions
From Glossary of Meteorology
No edit summary |
m (Rewrite with Template:Term and clean up) |
||
| Line 1: | Line 1: | ||
{{Term | |||
|Display title=amplitude | |||
{{ | |Definitions={{Definition | ||
|Num=1 | |||
|Meaning=Often the greatest magnitude at a given point of any spatially and temporally varying physical quantity governed by a [[wave equation]]; can also mean the spatial part of a time-harmonic [[wave]] function. | |||
|Explanation=For example, in the time-harmonic (or sinusoidal) [[scalar]] wave function with [[circular frequency]] ω, <blockquote>[[File:ams2001glos-Ae17.gif|link=|center|ams2001glos-Ae17]]</blockquote> where φ('''x''') is the (complex) amplitude of the wave, although the [[modulus]] of φ also may be called its amplitude. The (complex) amplitude of the scalar plane [[harmonic]] wave <blockquote>[[File:ams2001glos-Ae18.gif|link=|center|ams2001glos-Ae18]]</blockquote> with [[wavenumber]] ''k'' and initial [[phase]] θ is ''A'' exp(''ikx'' - ''i''θ), the modulus of which, [[File:ams2001glos-Aex03.gif|link=|ams2001glos-Aex03]], is also called the amplitude of the wave. In its most general sense, amplitude means extent or size. Thus the amplitude of a wave is some measure of its size. | |||
}} | |||
= | }} | ||
Latest revision as of 22:22, 27 March 2024
Often the greatest magnitude at a given point of any spatially and temporally varying physical quantity governed by a wave equation; can also mean the spatial part of a time-harmonic wave function.
For example, in the time-harmonic (or sinusoidal) scalar wave function with circular frequency ω,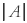 
, is also called the amplitude of the wave. In its most general sense, amplitude means extent or size. Thus the amplitude of a wave is some measure of its size.
where φ(x) is the (complex) amplitude of the wave, although the modulus of φ also may be called its amplitude. The (complex) amplitude of the scalar plane harmonic wave
with wavenumber k and initial phase θ is A exp(ikx - iθ), the modulus of which,
, is also called the amplitude of the wave. In its most general sense, amplitude means extent or size. Thus the amplitude of a wave is some measure of its size.
