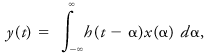convolution

Mathematical operation that is used to describe the imperfect response or resolution of an instrument or a measurement.

For example, the time response of a linear system to an input function is described by

where x(t) is the input function, y(t) the output, h(t) the weighting function characterizing the system, and α a variable of integration. The output is said to be the convolution of the input with the weighting function. Many instruments, because of limited frequency response, have the effect of smoothing the input data to produce an output that is more limited than the input in its frequency content. This effect is more readily understood by taking the Fourier transform of the convolution equation, in effect transforming from the time domain to the frequency domain. The result is

where Y, H, and X denote, respectively, the Fourier transforms of y, h, and x. The function H(f) is called the frequency response function of the system. The magnitude of H(f) determines whether frequency components that are present in the input function will also be present in the output or will be attenuated by the system. An example of a convolution process in radar is the smoothing of the spatial pattern of reflectivity as a consequence of the finite size of the pulse volume. Spatial irregularities with scales smaller than the pulse volume are attenuated in the measurement process by a convolution of the reflectivity field with the pulse volume.