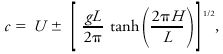gravity wave
From Glossary of Meteorology
(Also called gravitational wave.) A wave disturbance in which buoyancy (
or reduced gravity) acts as the restoring force on parcels displaced from hydrostatic equilibrium.
There is a direct oscillatory conversion between potential and kinetic energy in the wave motion. Pure gravity waves are stable for fluid systems that have static stability. This static stability may be 1) concentrated in an interface or 2) continuously distributed along the axis of gravity. The following remarks apply to the two types, respectively. 1) A wave generated at an interface is similar to a surface wave, having maximum amplitude at the interface. A plane gravity wave is characteristically composed of a pair of waves, the two moving in opposite directions with equal speed relative to the fluid itself. In the case where the upper fluid has zero density, the interface is a free surface and the two gravity waves move with speeds
see capillary wave). 2) Heterogeneous fluids, such as the atmosphere, have static stability arising from a stratification in which the environmental lapse rate is less than the process lapse rate. The atmosphere can support short internal gravity waves and long external gravity waves. The short waves (of the order of 10 km) have been associated, for example, with lee waves and billow waves. Such waves have vertical accelerations that cannot be neglected in the vertical equation of perturbation motion. The long gravity waves, moving relative to the atmosphere with speed ±(gH)½, where H is the height of the corresponding homogeneous atmosphere, have small vertical accelerations and are therefore consistent with the quasi-hydrostatic approximation. In neither type of gravity wave, however, is the horizontal divergence negligible. For meteorological purposes in which neither type is desired as a solution, for example, numerical forecasting, they may be eliminated by some restriction on the magnitude of the horizontal divergence. The above discussion is based upon the method of small perturbations. In certain special cases of water waves, for example, the Gerstner wave or the solitary wave, a theory of finite-amplitude disturbances exists.
See shear-gravity wave.
Gill, A. E. 1982. Atmosphere–Ocean Dynamics. Academic Press, . 95–188.
There is a direct oscillatory conversion between potential and kinetic energy in the wave motion. Pure gravity waves are stable for fluid systems that have static stability. This static stability may be 1) concentrated in an interface or 2) continuously distributed along the axis of gravity. The following remarks apply to the two types, respectively. 1) A wave generated at an interface is similar to a surface wave, having maximum amplitude at the interface. A plane gravity wave is characteristically composed of a pair of waves, the two moving in opposite directions with equal speed relative to the fluid itself. In the case where the upper fluid has zero density, the interface is a free surface and the two gravity waves move with speeds
where U is the current speed of fluid, g the acceleration of gravity, L the wavelength, and H the depth of the fluid. For deep-water waves (or Stokesian waves or short waves), H >> L and the wave speed reduces to
For shallow-water waves (or Lagrangian waves or long waves), H << L, and
All waves of consequence on the ocean surface or interfaces are gravity waves, for the surface tension of the water becomes negligible at wavelengths of greater than a few centimeters (
see capillary wave). 2) Heterogeneous fluids, such as the atmosphere, have static stability arising from a stratification in which the environmental lapse rate is less than the process lapse rate. The atmosphere can support short internal gravity waves and long external gravity waves. The short waves (of the order of 10 km) have been associated, for example, with lee waves and billow waves. Such waves have vertical accelerations that cannot be neglected in the vertical equation of perturbation motion. The long gravity waves, moving relative to the atmosphere with speed ±(gH)½, where H is the height of the corresponding homogeneous atmosphere, have small vertical accelerations and are therefore consistent with the quasi-hydrostatic approximation. In neither type of gravity wave, however, is the horizontal divergence negligible. For meteorological purposes in which neither type is desired as a solution, for example, numerical forecasting, they may be eliminated by some restriction on the magnitude of the horizontal divergence. The above discussion is based upon the method of small perturbations. In certain special cases of water waves, for example, the Gerstner wave or the solitary wave, a theory of finite-amplitude disturbances exists.
See shear-gravity wave.
Gill, A. E. 1982. Atmosphere–Ocean Dynamics. Academic Press, . 95–188.